Stunning Info About How To Tell If A Curve Is Smooth Matlibplot Line

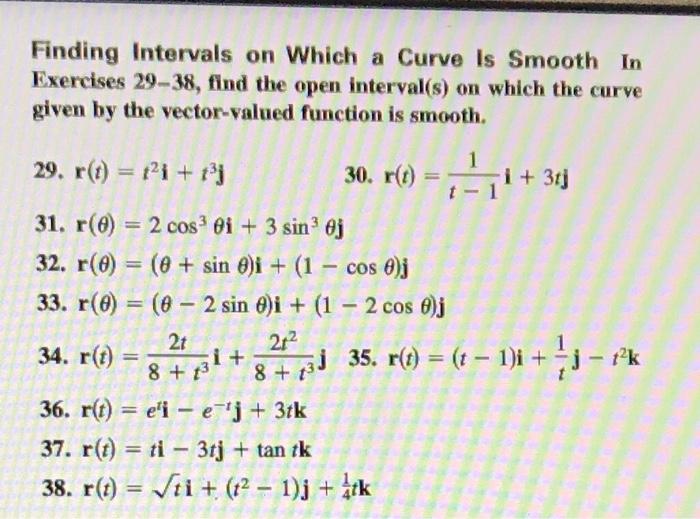
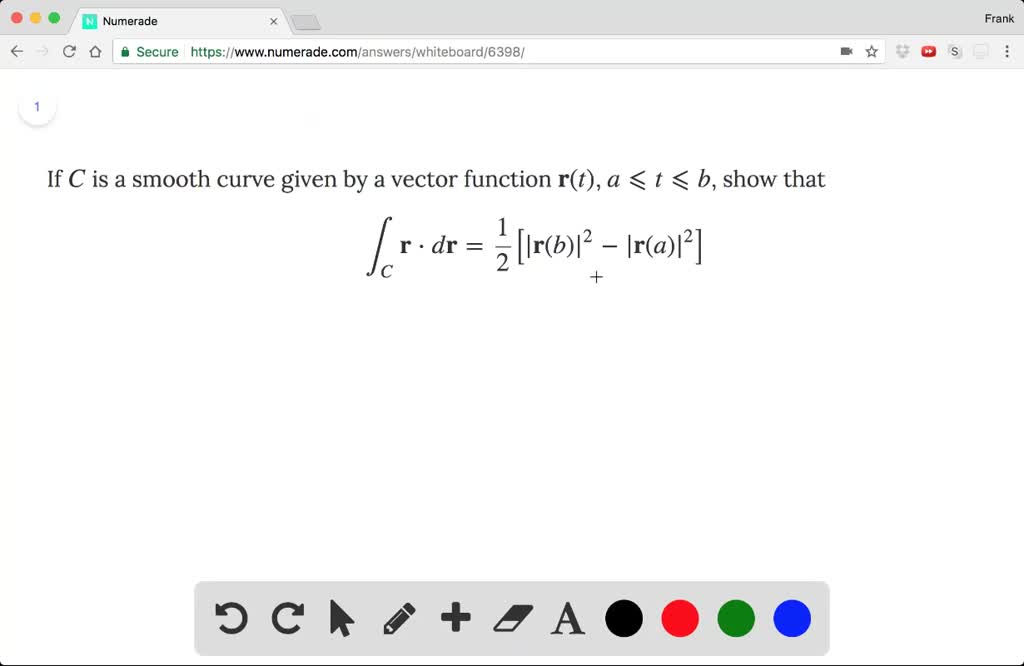
In this section we want to briefly discuss the curvature of a smooth curve (recall that for a smooth curve we require →r ′(t) r → ′ ( t) is continuous and →r ′(t) ≠ 0 r.

How to tell if a curve is smooth. Here r 0 (t) = 2i 1j + 1k which is Choose all answers that apply: For an algebraic curve of degree n, with , the curve is smooth in the real (resp.

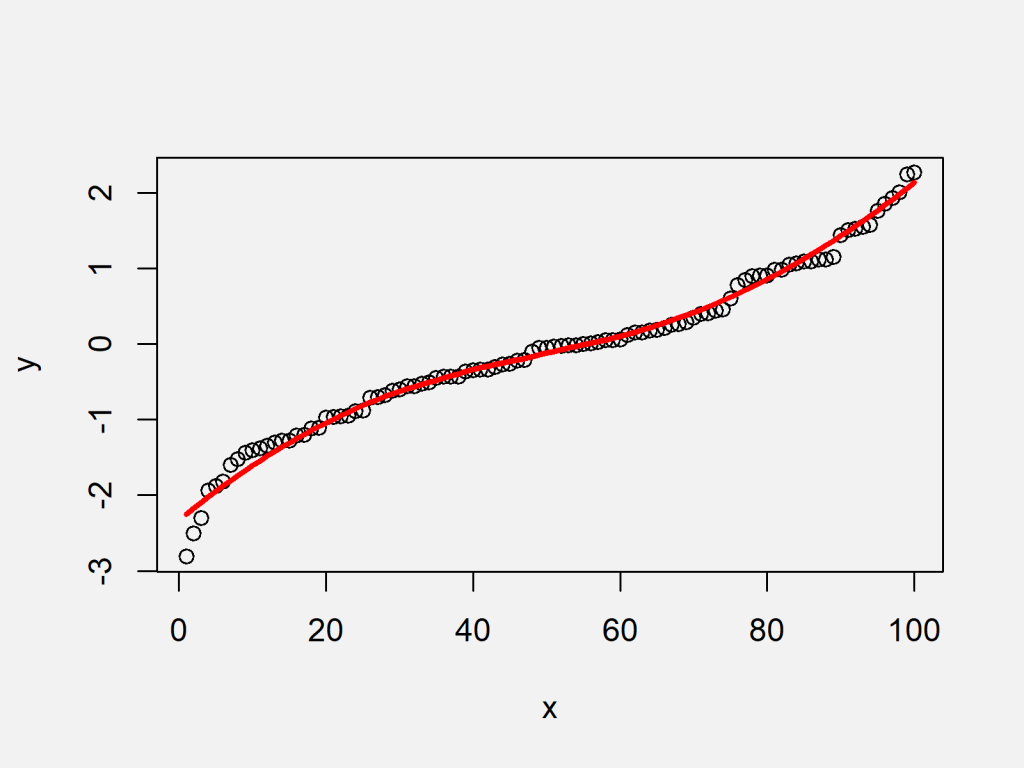
The main issue is that second (and other) derivatives are dependent on the parameterization of the curve, whereas smoothness is a geometric property that is. I'd like an automatic way to find the best smoothing factor s for a spline fit to a given set of data points. A curve $\mathbf{r}(t)$ is considered to be smooth if its derivative, $\mathbf{r}'(t)$, is continuous and nonzero for all values of $t$.

A curve is piecewise smooth if it has a piecewise smooth parametrization. In this video, i show that a curve described by a vector function is not smooth by showing there are values of t that make the derivative equal to zero. Is the curve defined by:

A smooth curve α: My logic says yes because one can break it into a finite number of smooth. It intersects itself, has a cusp.

The terms parametric continuity (c k) and geometric continuity (g n) were introduced by brian barsky, to show that the smoothness of a curve could be measured by removing. R(t) = (2t+ 1)i + (3 t)j + tj for all t. A curve can fail to be smooth if:

The algorithm for identifying whether or not a parametric curve is smooth contains a first step of finding out when dx/dt and dy/dt is simultaneously 0. 2 4 − 2 − 4 2 4 − 2 − 4 y x. Equivalently, we say that α is an immersion of i into r3.

Consider the following curve in the plane, $(x(t),y(t))$, this curve is called smooth if the functions $x(t)$ and $y(t)$ are smooth, which simply means that for all $n$, the derivatives $\frac{d^nx}{dt^n}$ and $\frac{d^ny}{dt^n}$ exist. Here's a sample visualization of some data and the fit. A smooth curve is a curve which is a smooth function, where the word curve is interpreted in the analytic geometry context.

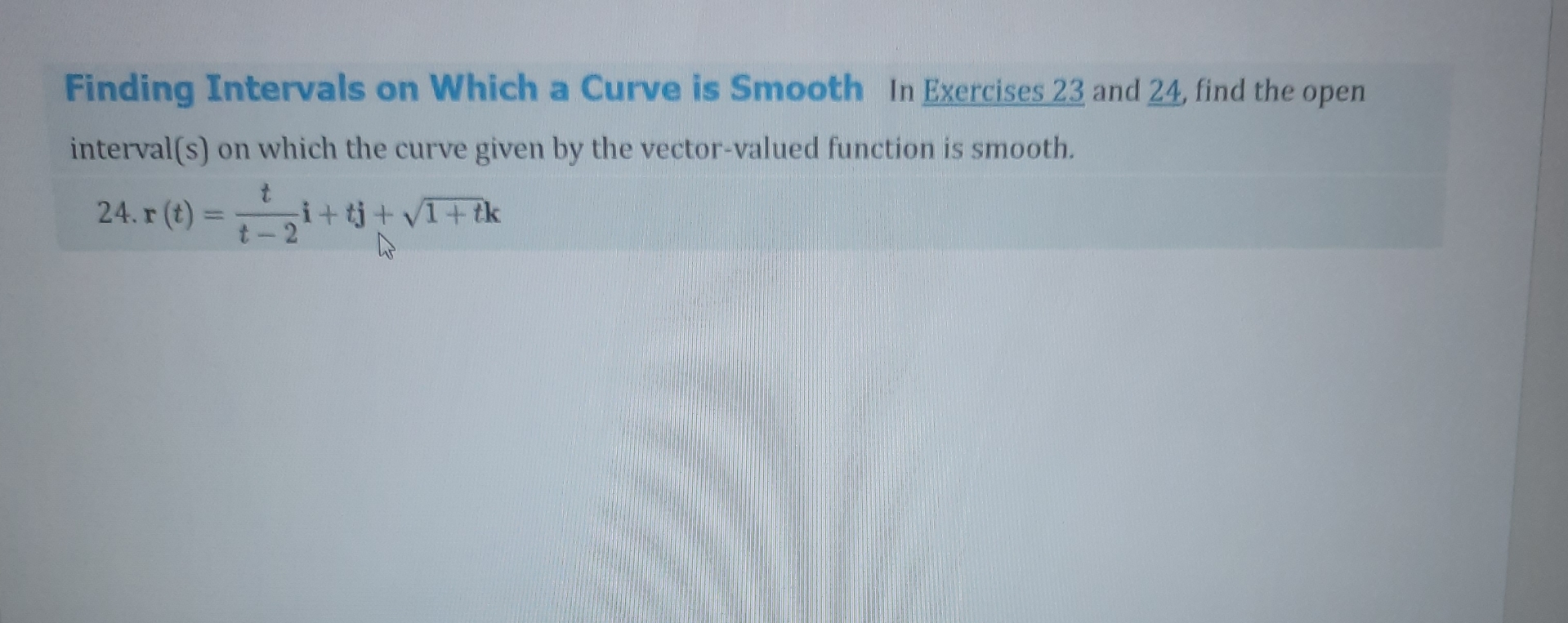
A curve is smooth if every point has a neighbourhood where the curve is the graph of a differentiable function. Complex) projective plane if the system has no other real (resp. I have to determine whether the following curves are smooth or not and i'm having trouble with the following two functions:

In particular, a smooth curve is a. Complex) solution than (0, 0, 0). Here's a curve s :

The curve α(t) = (t3, t2) in the plane fails to be. We define the positive direction on a contour \(c\) to be the direction on the curve corresponding to. This video explains how to determine a piecewise smooth parameterization of a curve made up of a line segment and square root function.