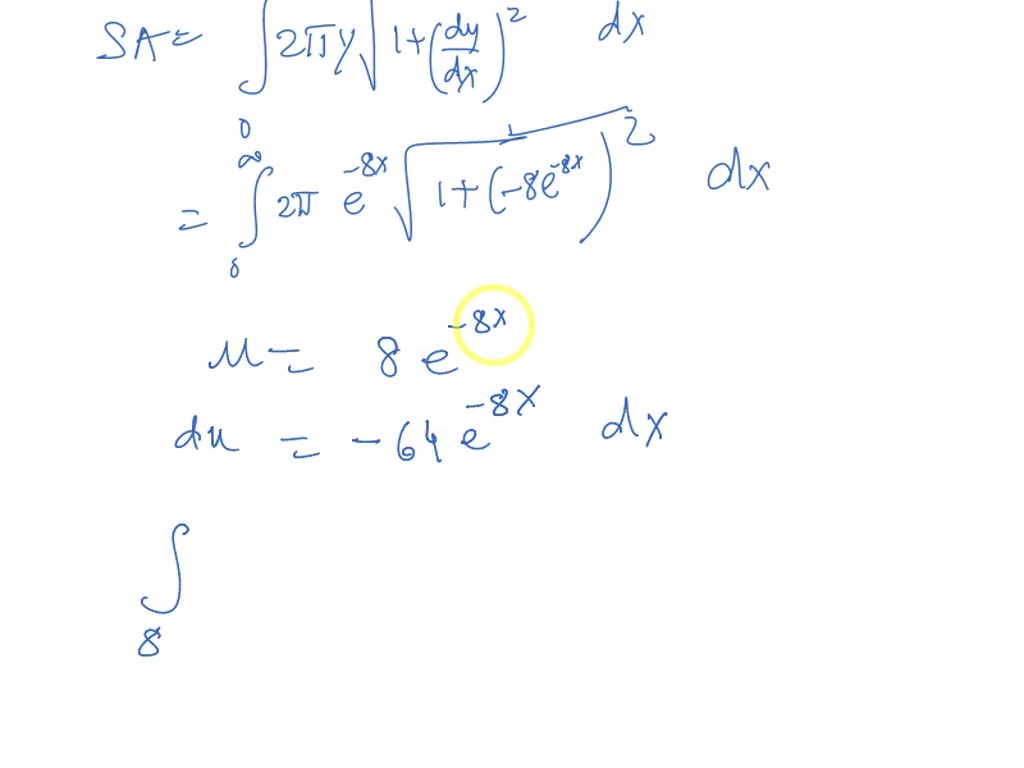Here’s A Quick Way To Solve A Tips About Is A Curve Infinite Vuetify Line Chart

I have not learned how to solve infinite regions and.

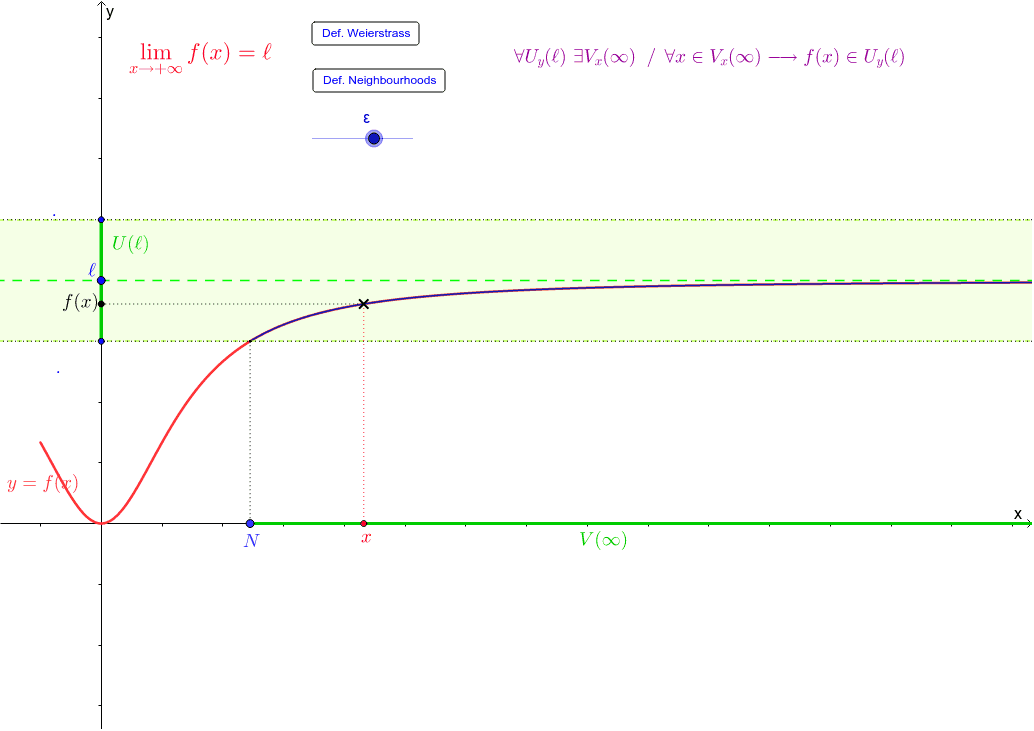
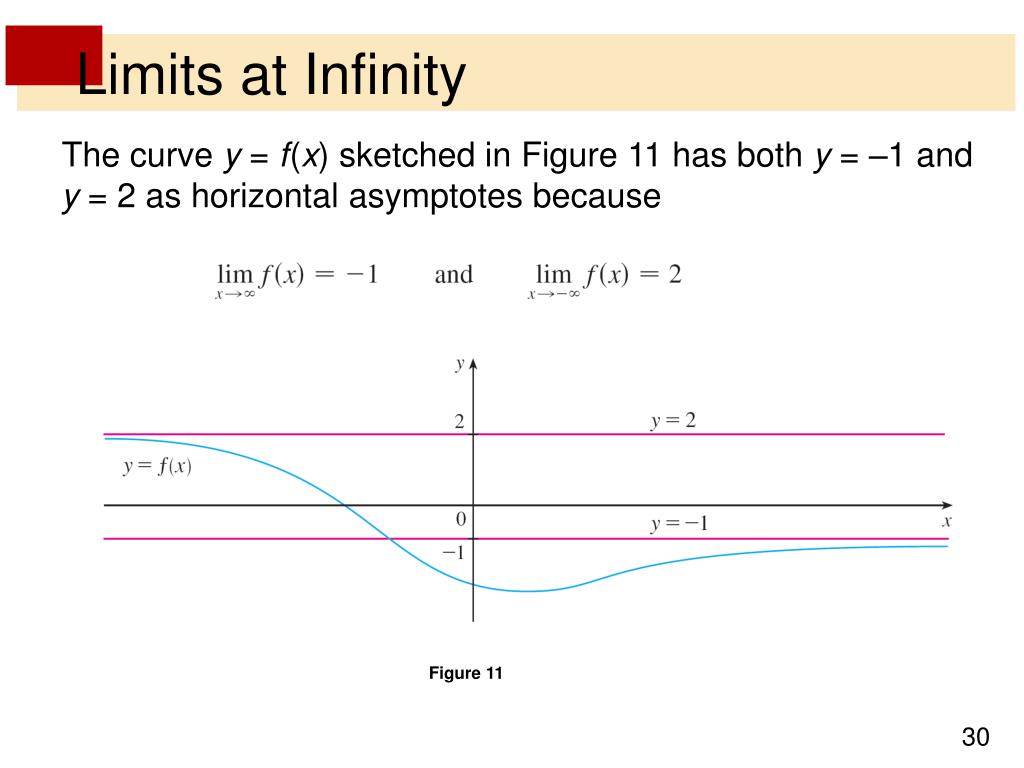
Is a curve infinite. I am trying to find the area between two curves for this problem. In other words, the curve and its asymptote get infinitely close, but they never meet. What does it look like if $\kappa(t)=\infty$?

Suppose that the curve tends to infinity, that is: Since there are infinitely many wiggles and each of them contributes a curve. However, at the point where $t=0$, there is a sharp corner in the curve, and.

But one infinite set can completely contain another and they can still be the same size, kind of the way “infinity plus 1” isn’t actually a larger amount of love than. The statement that a line is an. Intuitively, a curve may be thought of as the.

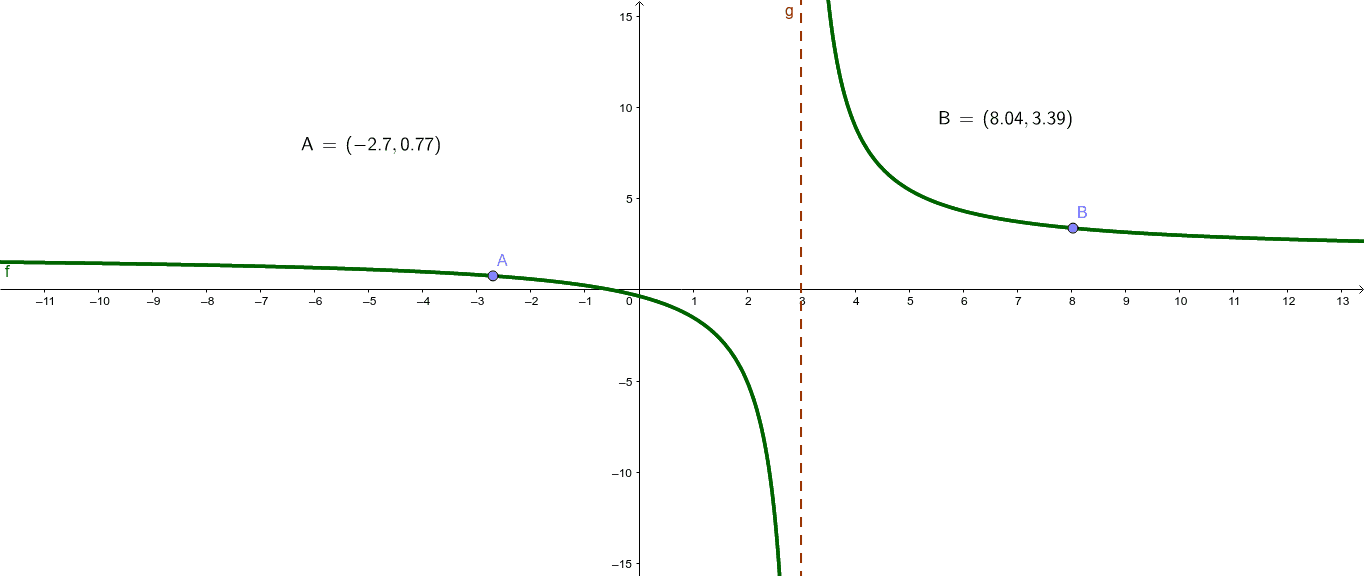
The curvature of the universe places constraints on the topology. A point at infinity of the curve is a zero of p of the form (a, b, 0). Asymptotes have a variety of.

The simplest way to see this is probably to notice that the curve length between each maximum and the neighboring minimum must be at least $2$. From the definition, only open curves that have some infinite branch can have an asymptote. For example, $\kappa(t)=0$ means that the curve is kind of flat at that point.

Clearly this curve is $c_\infty$. X = 2 x = 2. The limit is a horocycle, a curve of constant and nonzero curvature which does not close up to form a circle, but.

Essentially, there are only two kinds of curves of constant curvature: Equivalently, (a, b) is a zero of p d. Y = 1 x2 y = 1 x 2.

Infinite craft is a game whose title is almost literal. On desktop, continuous scrolling search results will be replaced by a pagination bar and, on mobile, a “more results” button. A straight line the distance of which from the point $ (x, f (x)) $ on the curve tends to zero as the point moves along the branch of the curve to infinity.

Does it always correspond a corner? Informally, such curves are said to have infinite length. The koch curve has an infinite length, because the total length of the curve increases by a factor of with each iteration.

The fundamental theorem of algebra implies that, over an algebraically closed. Each iteration creates four times as many line segments as. Fractals are useful in modeling structures (such as eroded coastlines or snowflakes) in which similar patterns recur at progressively smaller scales, and in describing partly.