Amazing Info About What Is Correlation In Line Of Best Fit Finding The Tangent At A Point

Give the equation in its simplest form.

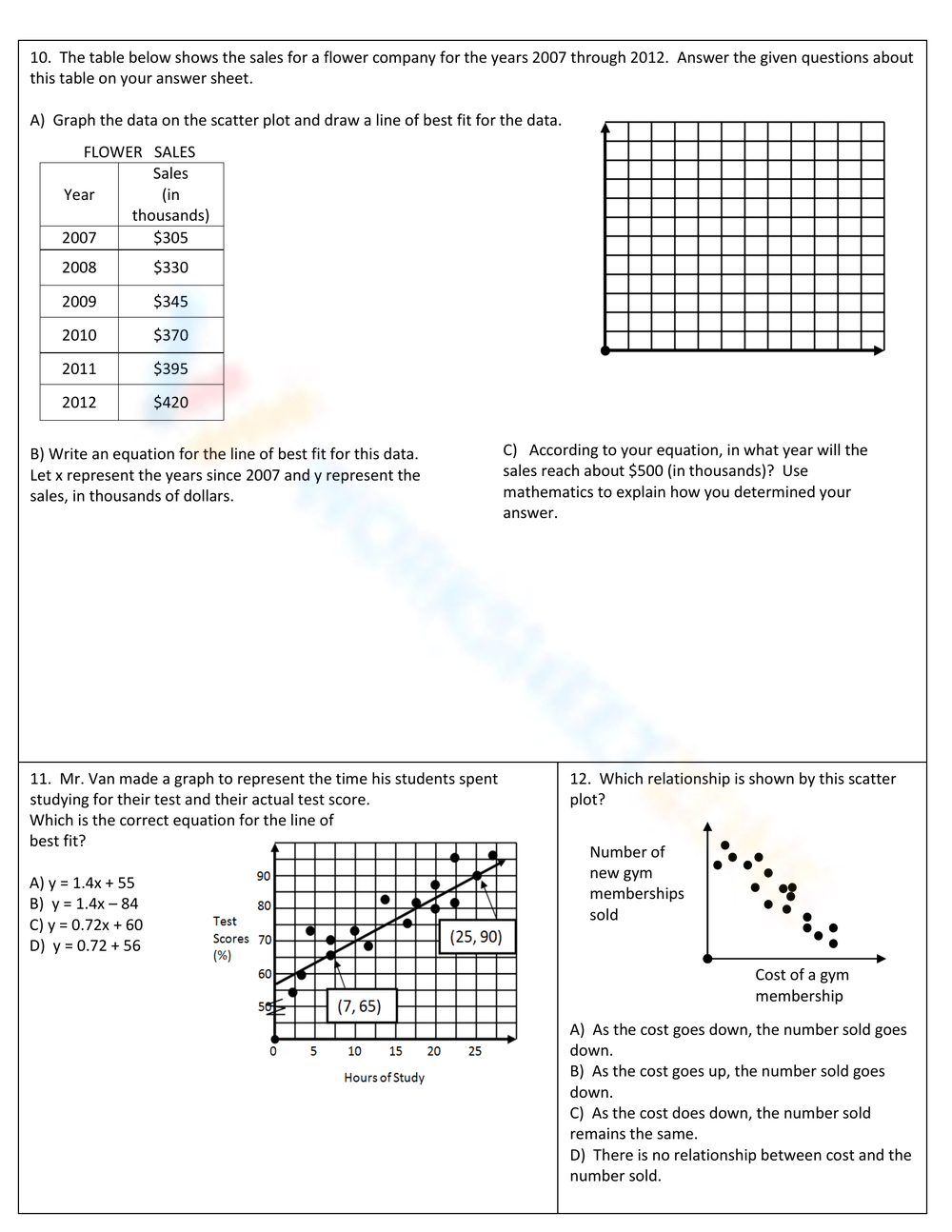
What is correlation in line of best fit. Beginning with straight lines; Estimating equations of lines of best fit, and using them to make predictions. Y ^ i = b 0 + b 1 x i.

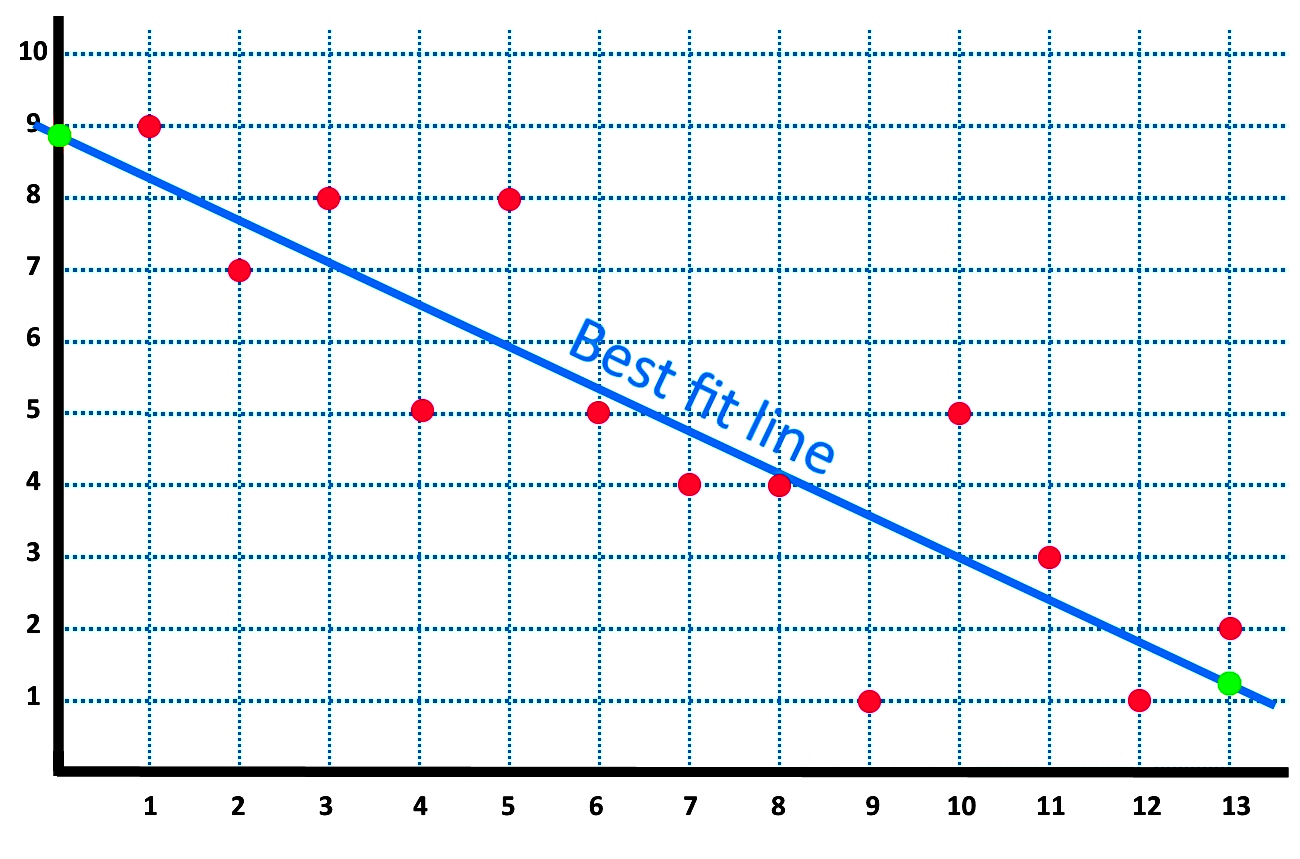
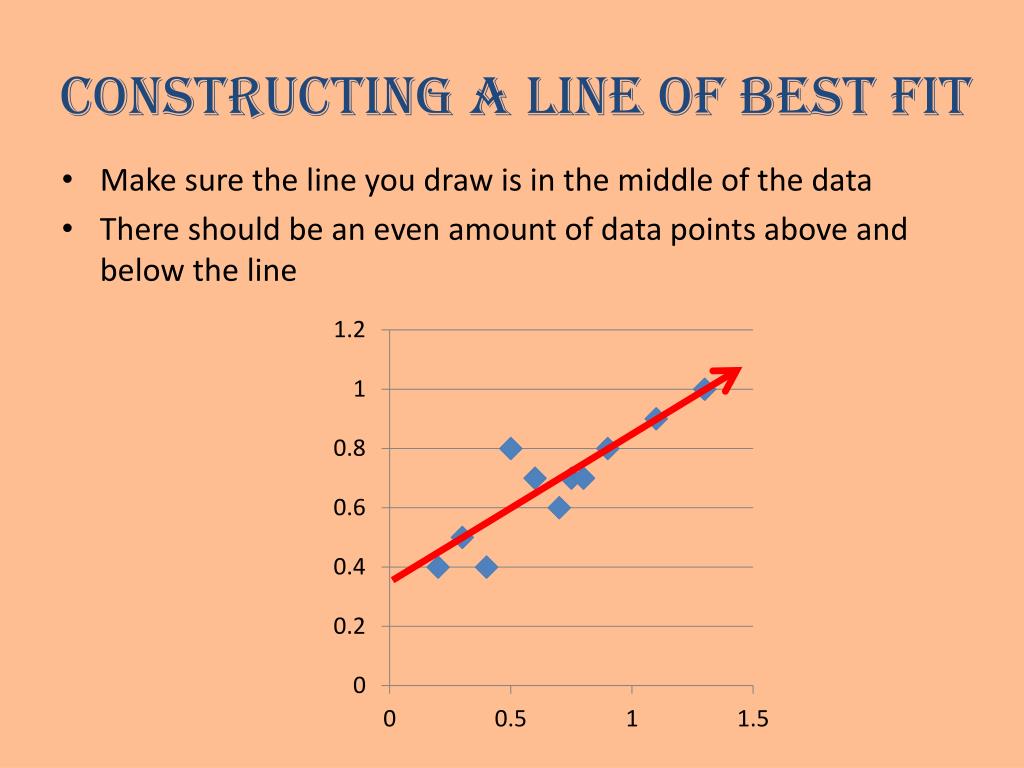
It is referred to as an estimated line of best fit because it is drawn by hand following some guidelines. \[y=0.458 x+1.52 \nonumber \] we can superimpose the plot of the line of best fit on our data set in two easy steps. This can then be used to make predictions.

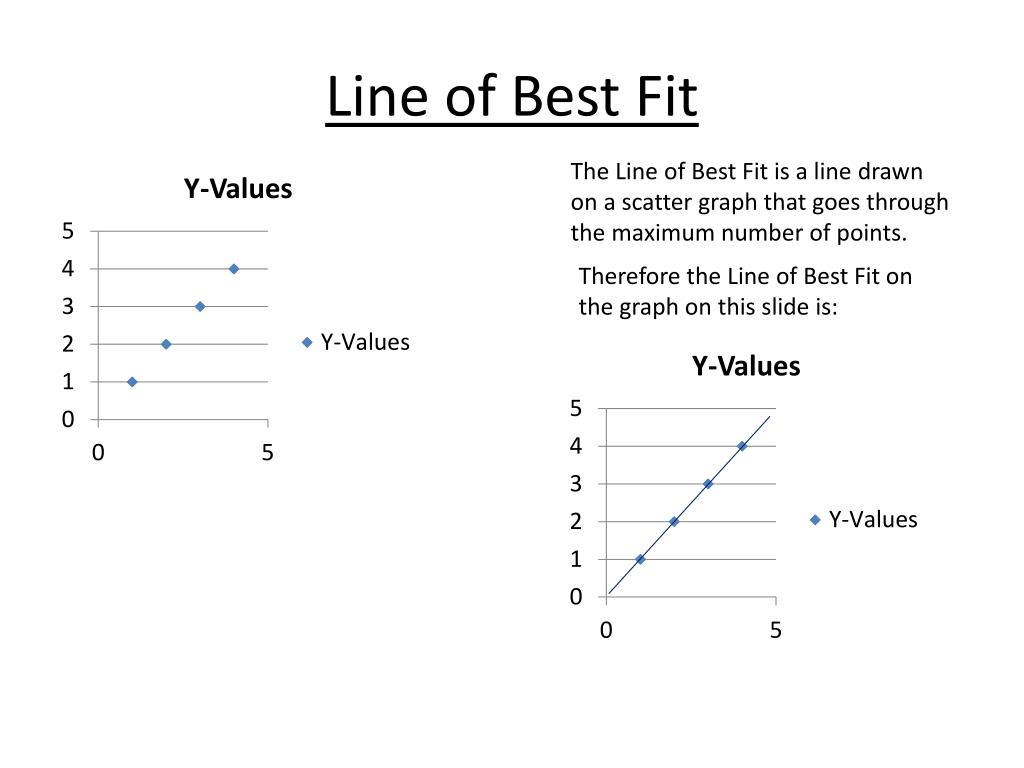
You need to be able to find the mean point, to draw a line of best fit. The equation of the best fitting line is: The equation of the best fitting line is:

(b) use your answer to part (a) to estimate the value of q when p = 4. A line was fit to the data to model the relationship. It can be depicted visually, or as a mathematical.

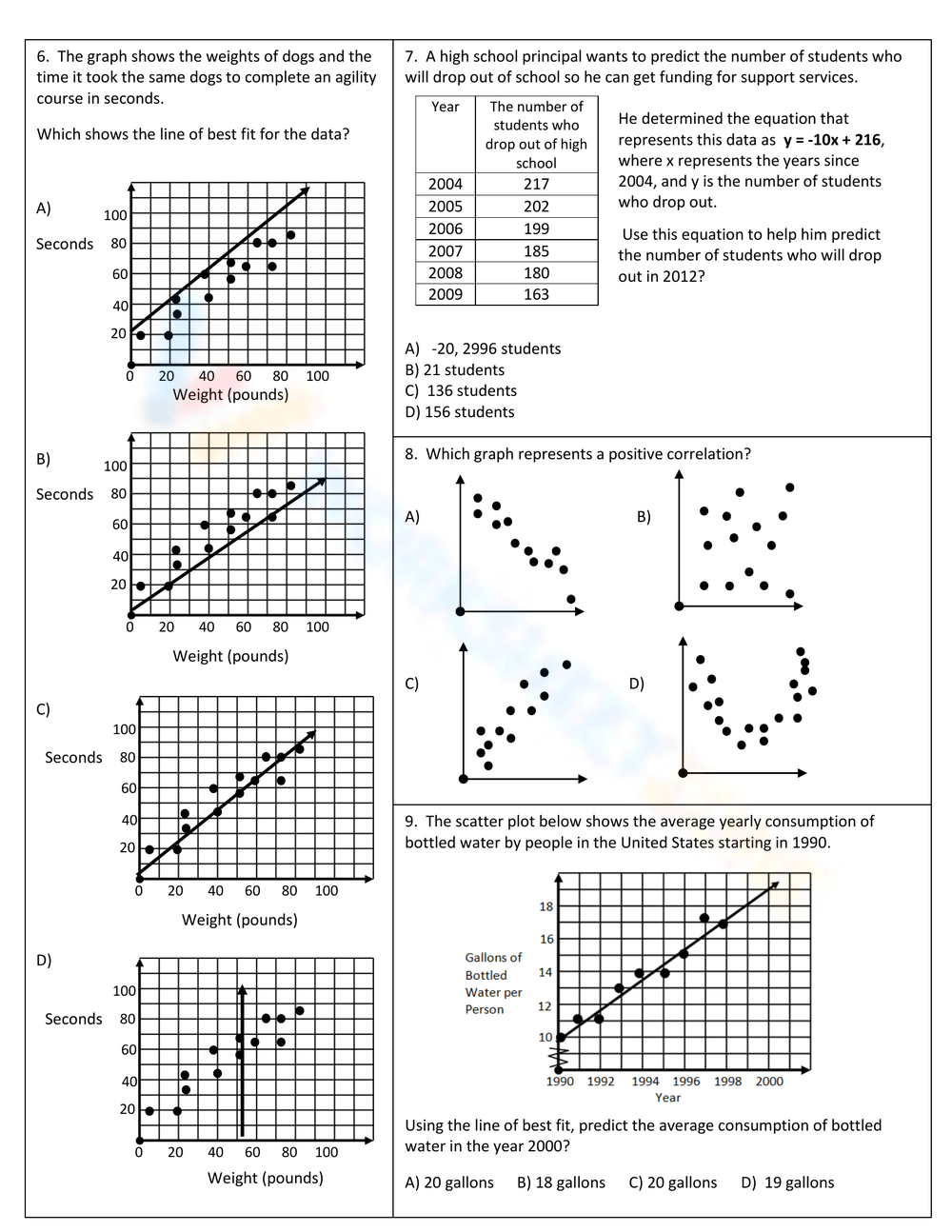
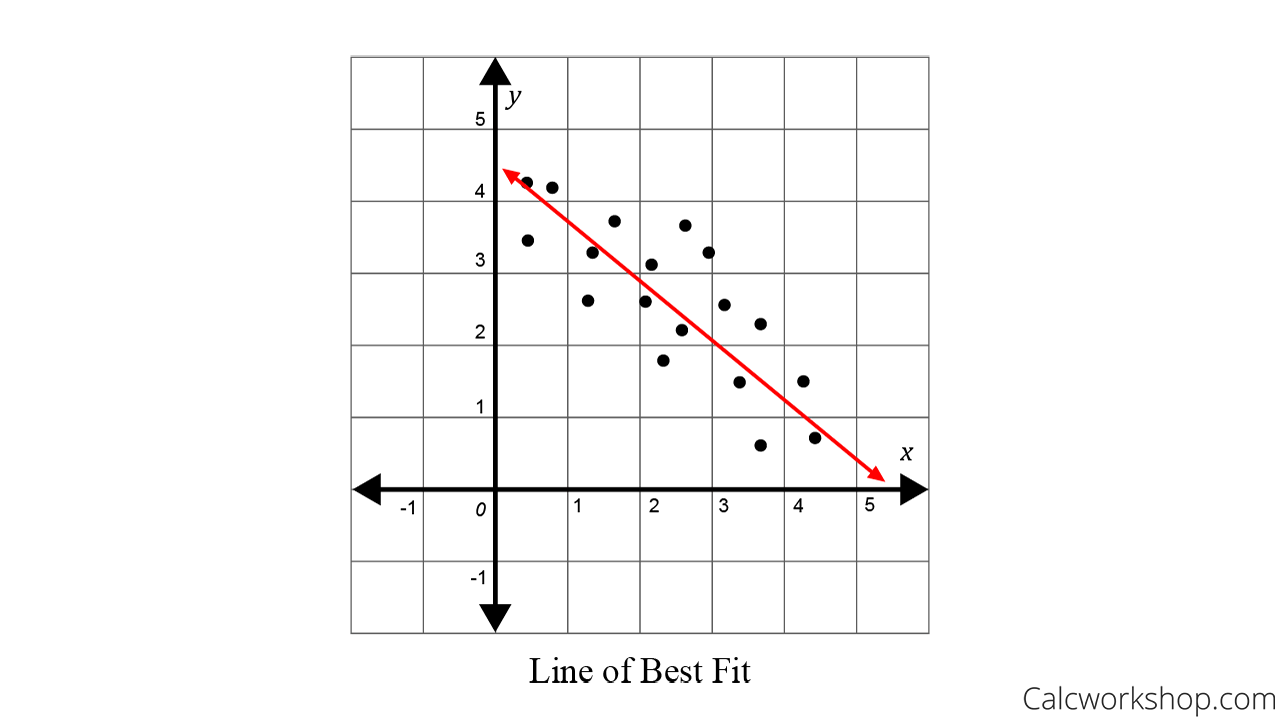
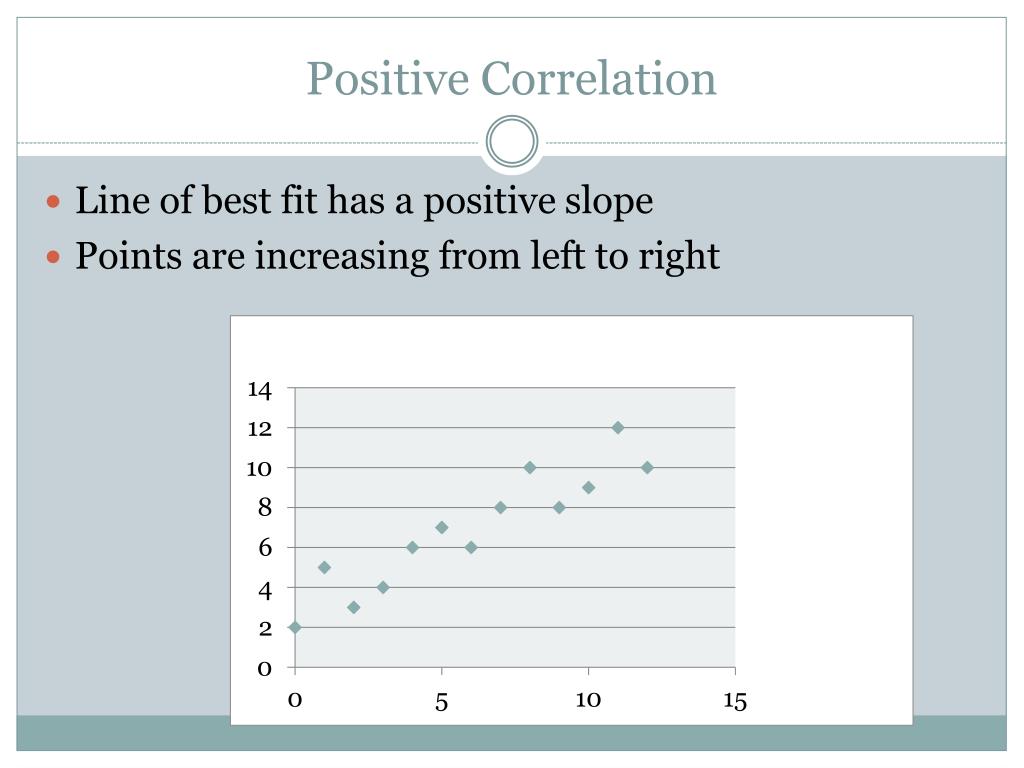
To create categories within the data. Each celebrating a chapter in the artist's musical journey. If you have a linear relationship, you’ll draw a straight line of best fit that takes all of your data points into account on a scatter plot.

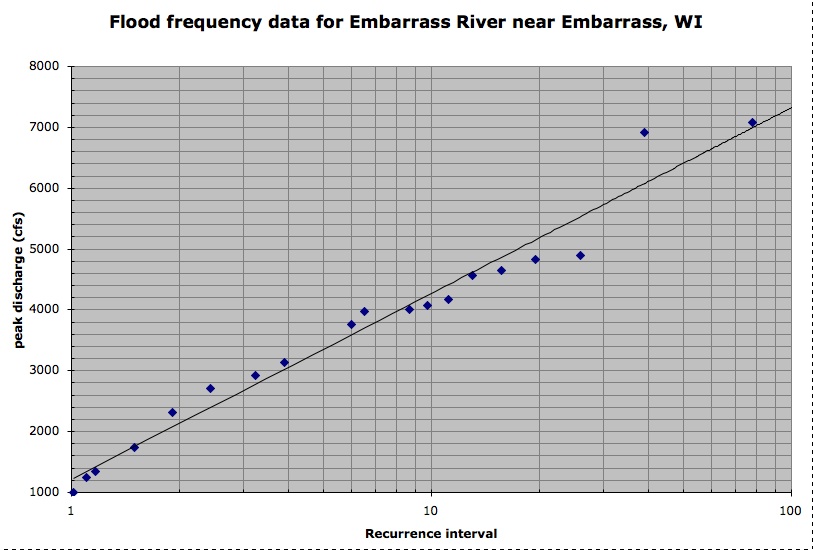
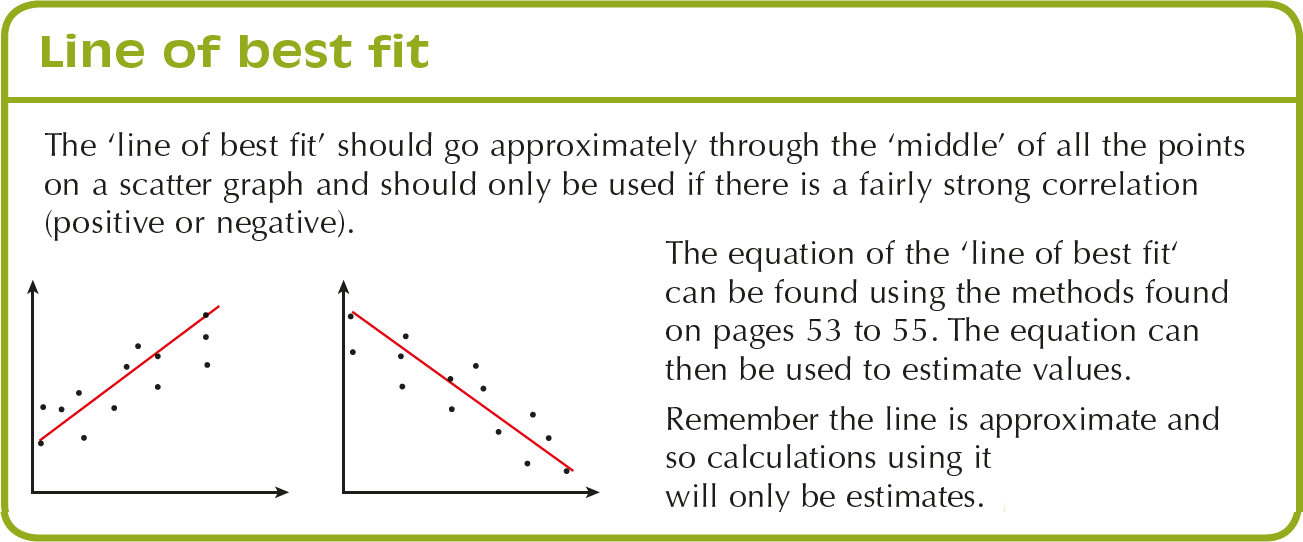
A regression line, or a line of best fit, can be drawn on a scatter plot and used to predict outcomes for the \(x\) and \(y\) variables in a given data set or sample data. Does arcgis experience builder have any widget that can do statistical correlation like make a line of best fit. The line of best fit can be defined as an intelligent guess or approximation on a set of data aiming to identify and describe the relationship between given variables.

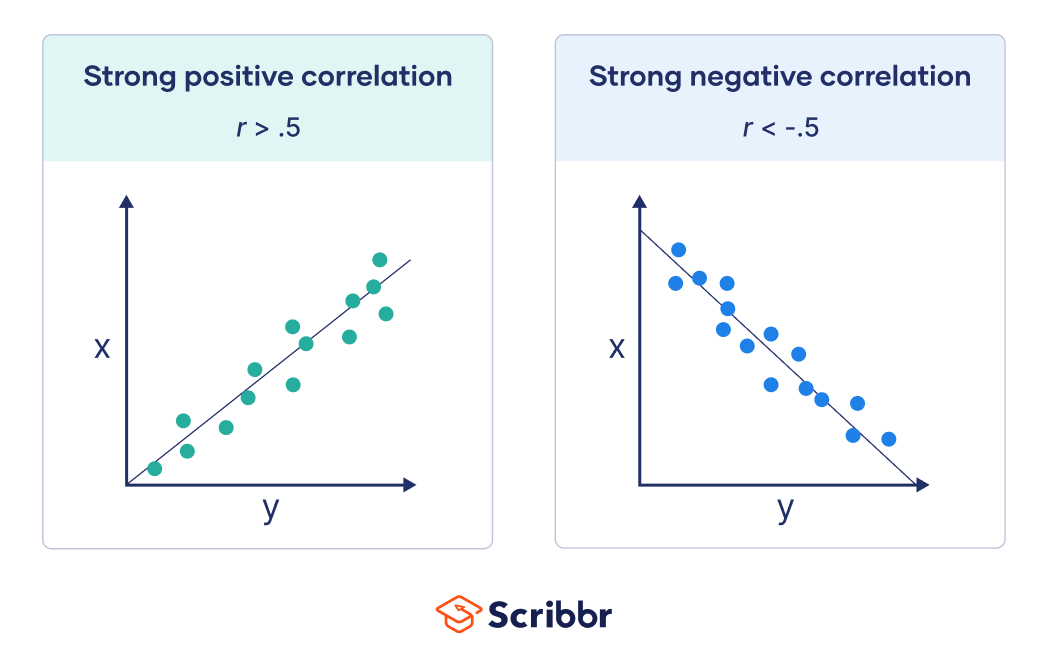
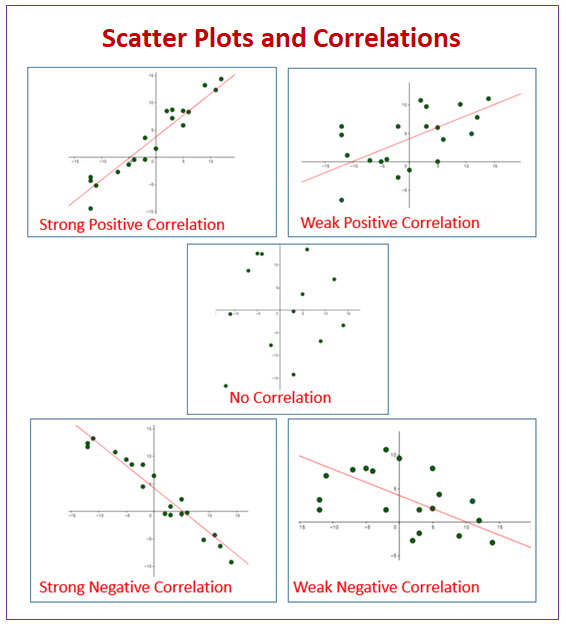
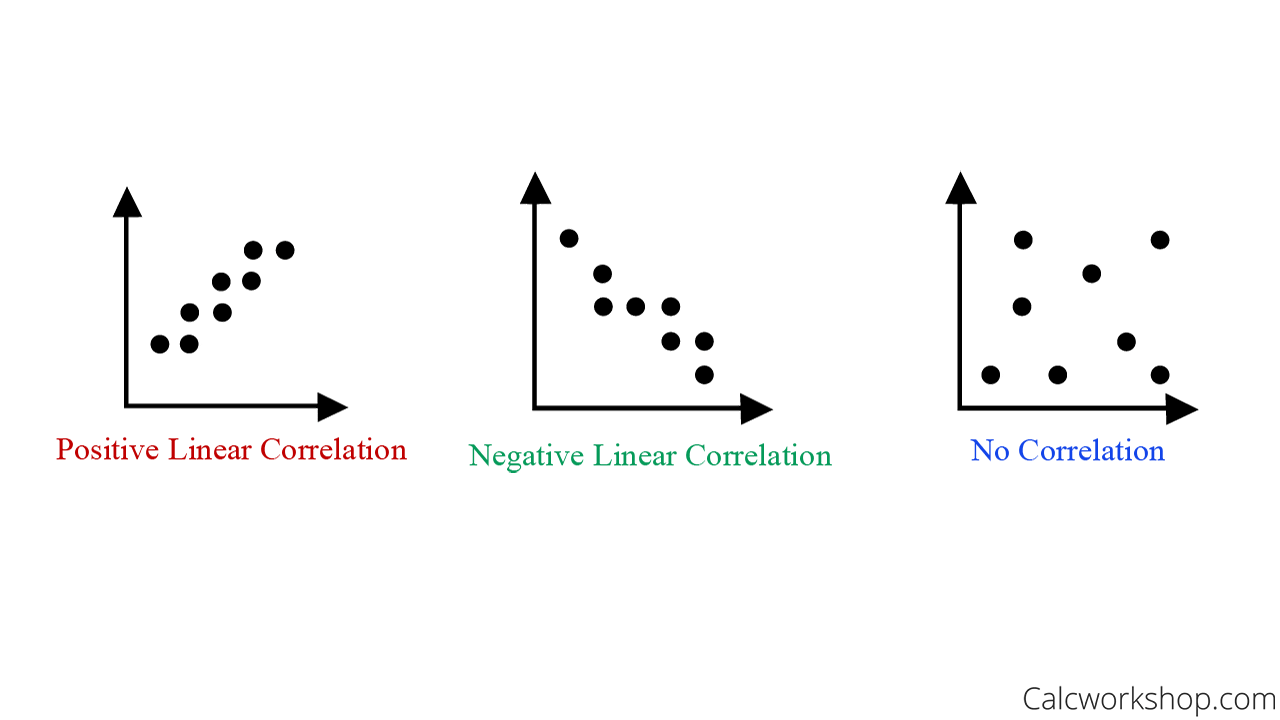
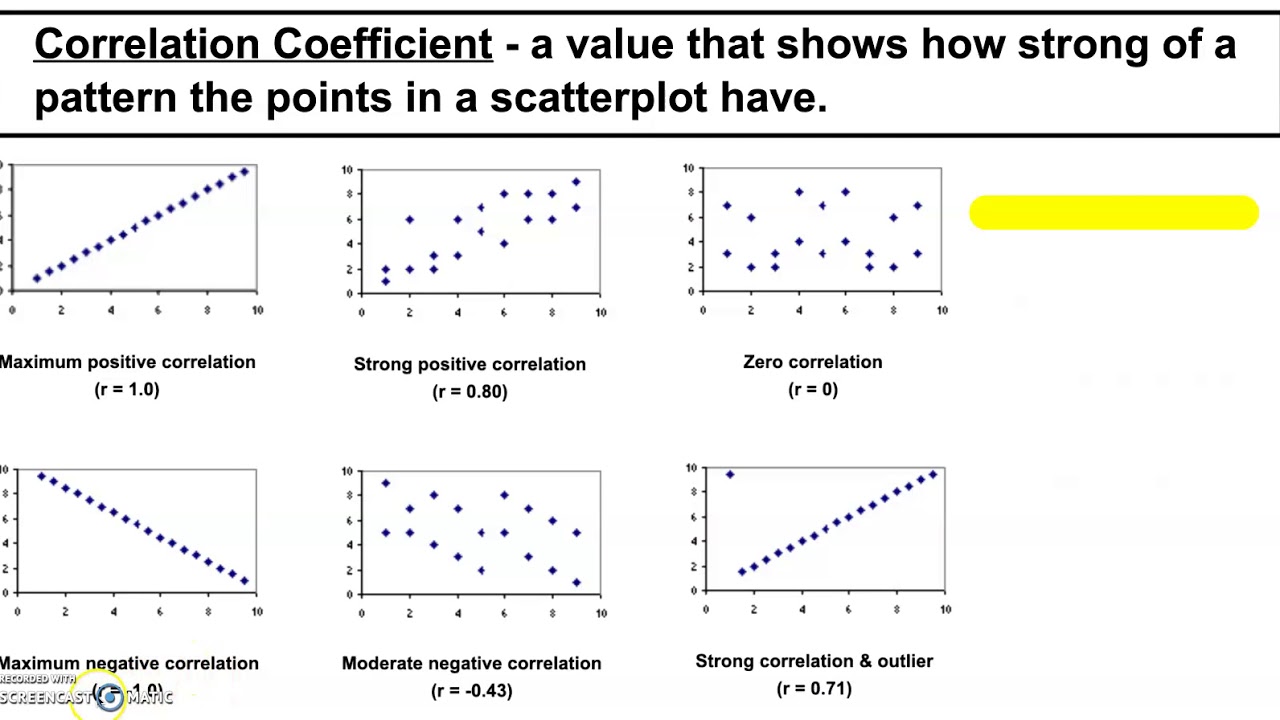
The correlation coefficient tells you how closely your data fit on a line. The sum of squared errors, when set to its minimum, calculates the points on the line of best fit. The linear regression model attempts to find the relationship between variables by finding the best fit line.

Here's how you might think about this quantity q: What is a line of best fit? In statistics, it is a type.

(c) estimate the value of p when q = 3. This can then be used to make predictions. A line of best fit can often be drawn by eye.

In the context of bivariate numerical data, what is the purpose of the line of best fit? To measure the spread of the data.